symmetries IV (plain plane)
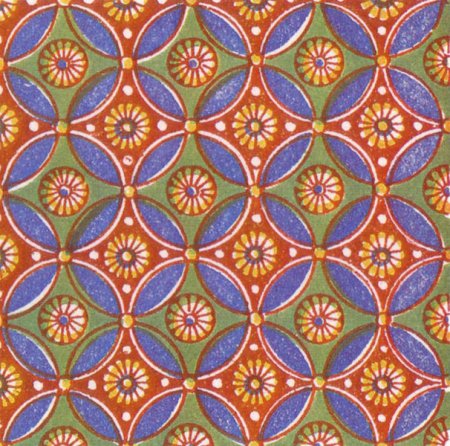
A classic topic in intersections between math and art/design are tesselations. This is mostly due to the fact that the included math is almost completely visualizable, which can’t be said for most parts of mathematics.
A tessellation or tiling of the plane is a collection of plane figures that fills the plane with no overlaps and no gaps. I.e. the figures are arranged in “some periodic” fashion in order to cover the plane – just as real tiles. In the regular case – due to some periodicity – thewhole plane could be transformed (e.g. shifted by a certain distance) and the pattern would look the same. Such a transformation of the plane which leaves the pattern look as before (mathematicians say invariant) is called a symmetry. Likewise one could use a symmetry in order to arrange the figures in the right order: start with one basic figure on a infinetely big canvas (the plane), apply the transformation (e.g. shift the plane) and merge the two canvases into one so that you have two figures now a.s.o. Mathematicians call this process of creating a pattern: a pattern is generated by the given symmetries. A pattern which is generated with shifts, rotations and reflections is called a wallpaper pattern, because it looks like a wallpaper -also if this procedure is also used for floors :-):

There exist 17 symmetries with which all existing “wallpaper patterns” can be generated. These 17 symmetries form a group the socalled wallpaper group. (Almost) all seventeen of these symmetries can be found in tilings in the Alhambra in Granada, Spain. (It seems that Coxeter disputed one of the examples in math reviews thats why I put the “almost”).
-A classic book covering tilings and patterns: Grunbaum, Branko and G. C. Shephard. Tilings and Patterns. New York: W. H. Freeman & Co., 1987. ISBN 0716711931.
-create your own tesselation with this tesselation applet
– a nice show of historic tilings can be found at this tiling site
-very funny are also these animated tessalations by
Makoto Nakamura (Many thanks to John for this link)
– the importance of seams in tilings is beautifully illustrated by the famouscaffee wall illusion
Tilings exist also in other spaces (like hyperbolic tilings), with sometimes other dimensions and tilings need not be “periodic”, like for the case of famous penrose tilings which are “quasiperiodic”. But this would be worth another post. However to play around with penrose tilings there is this nice penrose tilings applet
October 16th, 2006 at 7:57 pm
[…] In the randform post about tesselations it was already mentioned that there are not so many mathematical things which can be visualized easily or visualized at all. However next to the tesselations there is a very popular area of mathematical visualization which is Origami. Origami is an active field of mathematical research as well as of arts. […]
November 25th, 2013 at 12:58 am
Nice post. I learn something new and challenging on websites I stumbleupon on a daily basis. It’s always helpful to read through articles from other writers and use a little something from other web sites.