«
»

confocal quadrics

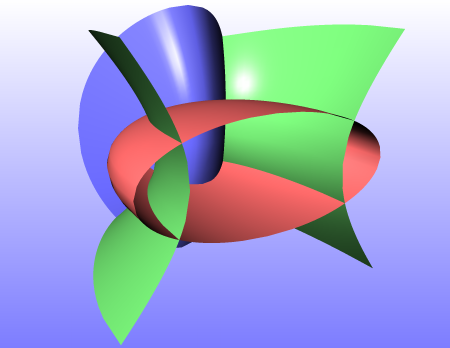
confocal.png

Confocal quadrics are known to form a triply orthogonal coordinate system on most of the three dimesional space.

This means there are three one parameter families of surfaces (in this case they are ellipsoids, hyperboloids of one sheet, and hyperboloids of two sheets) such that through every point there goes exactly one surface of each family and the surfaces intersect orthogonaly. Since this is sometimes difficult to imagine I made a webstart for it. The surfaces are cut in half so that one can better see what is happening, and in case of the hyperboloids they are cropped of course (these surfaces are unbounded). Three sliders let you browse through the three families.

posted by timh on Monday, December 11th, 2006 at 8:00 pm // math, visualization. // RSS 2.0 feed skip to the end and leave a response // pinging is currently not allowed //

One Response to “confocal quadrics”

  1. Burak Unveren Says:
    September 21st, 2008 at 8:57 pm

    that is a wonderful work. thanks a lot. nowadays, i study differential geometry and i needed some help to visualize how a triply orthogonal system looks like. your site is was handy. keep up the good work…

    p.s: by the way, unfortunately, i could not make the link “webstart” work.

Leave a Reply


The below box is for leaving comments. Interesting comments in german, french and russian will eventually be translated into english. If you write a comment you consent to our data protection practices as specified here. If your comment text is not too rude and if your URL is not clearly SPAM then both will be published after moderation. Your email adress will not be published. Moderation is done by hand and might take up to a couple of days.
you can use LaTeX in your math comments, by using the [latex] shortcode:
[latex] E = m c^2 [/latex]